Describe the Translation in Each Function

F x x2 f x x 2. Similarly we add 3 to x when we translate three units to the left.

Translation Rules Simply Explained W 5 Examples

5 The graph is translated ½ unit to the left.

. Gx x2 g x x 2. The parent function is the simplest form of the type of function given. This described the required translation.

Describe the translation in each function. The path of a diver diving off a 10-foot platform can be represented by the function hx -05x2 - 4x - 21 where h is the divers height abo. W x x3 4x 3.

Gx 2x 1. The period is given by the fraction 2ˇ b ie. By translating the function its graph is moved up an down.

Here are some things we can do. W x x3 4x 2. Translations The graph of a function can be moved up down left or right by adding to or subtracting from the output or the input.

Translations of the Common Functions. By Literature Title. The transformation from the first equation to the second one can be found by finding a a h h and k k for each equation.

To move vertically a constant is added. _____ PERIOD _____ Transformations of Linear Functions Practice Examples 1 through 3 Describe the translation in each function as it relates to the graph of the parent function. Translate f x h units to the right.

This shows that the function f x was translated to the left by 5 units to form g x. 1 Rewrite the equation by factoring -8 from the radicand and taking the cube root to get -2 in front of the radical symbol. Describe the Transformation f xx2.

Then graph the function. Move 4 spaces right. For example the function y sin2x does not have period 2.

165 reflection of the graph of y x2 across the x-axis 165 reflection of the graph of y x2 across the y-axis Describe the dilation in each function. Y 3 sqrt -8x - 4. These two processes are essential for life.

Then graph the function. Factor a 1 1 out of the absolute value to make the coefficient of x x equal to 1 1. Here are the graphs of y f x y f x 2 and y f x - 2.

4 4 units right and 2 units up fx tx. Wx 2x 1. Hx 2x 3.

Transformations are often easiest to analyze by focusing on how the location of specific points on the curve have changed. The graph of fx 2x is shown. F x x f x x gx x 5 g x x 5.

2 y -x8x y - x2-8x y - x- 4 2-16 Parent y 2 2 function y - 2 - 4 16 Horizontal translation 4 units right. 3 The graph is also reflected over the y-axis. 4 The graph is vertically stretched by a factor of 2.

Up to 24 cash back translation of the graph of y x down 3 units Describe the reflection in each function. Move 2 spaces up. When we translate a graph three units to the right we subtract 3 from the input variable x.

The table below generalizes horizontal transformations for all types of function f x. The transformation being described is from gx x2 g x x 2 to f x x2 f x x. Stretch it by 2 in the y-direction.

Yx2 4 Please give a detailed answer I dont understand 1 See answer Advertisement. Vertical translation up 16 units. Y x y.

Reflection over x-axis. A shift will move the graph to a new location on the coordinate system. Solution for Describe the translation in each function as it relates to its parent graph.

Up to 24 cash back Parent function. Sketch a graph of each function as. Translation is a function transformation that transform the function in a way of translating.

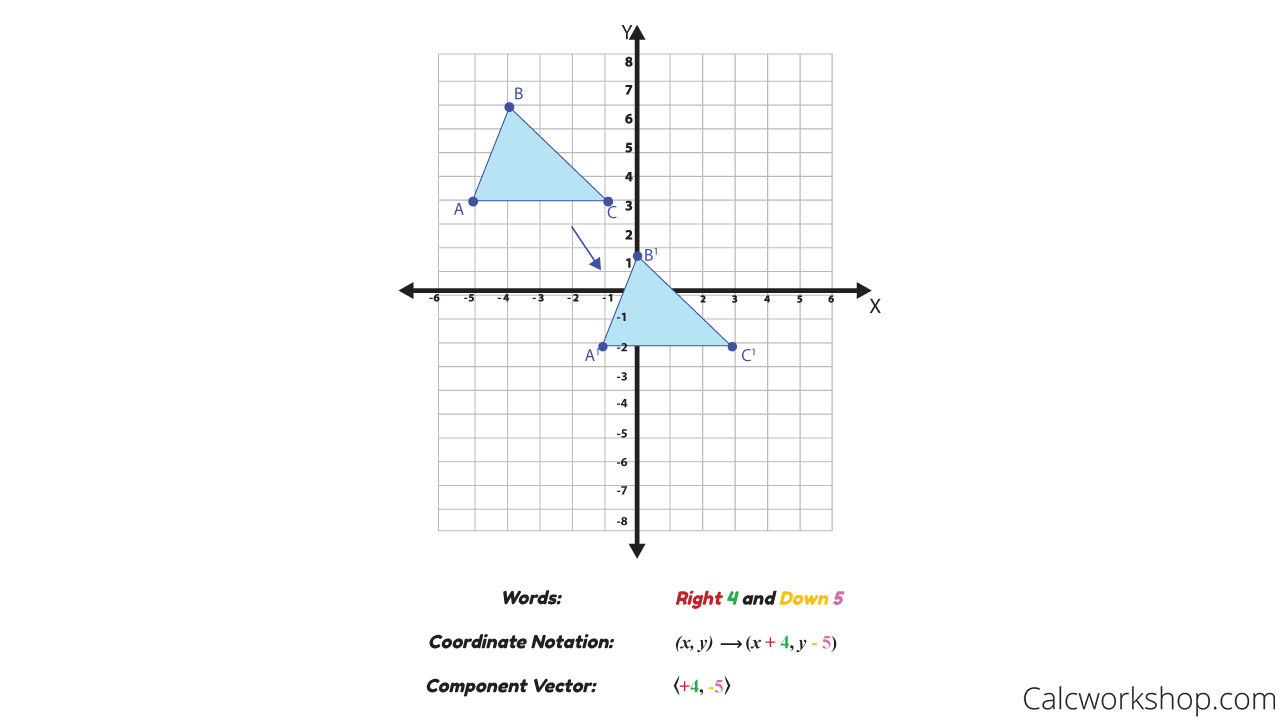
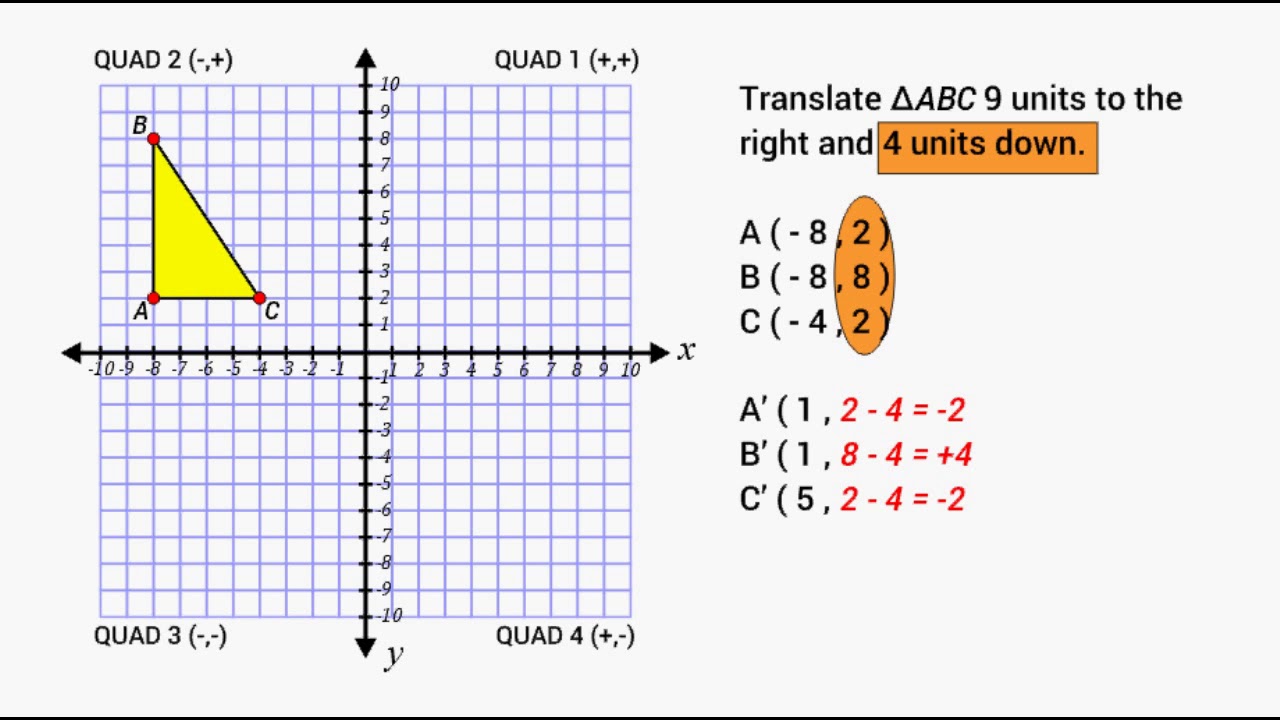
To describe a translation we need to say in which direction and by what distance each point is moved. Y x2 4. Reflection over X-axis.

Translation always involves either addition or subtraction and you can quickly tell whether it is horizontal or vertical by looking at whether the operation takes place within the parentheses of a function or is completely separate from the function. Using a Column Vector to Describe a Translation A column vector is used to describe a translation. NAME _ DATE_ PERIOD _ Transformations of Linear Functions Practice Examples 1 through.

Vertical translation up 4 units. They are found in all organisms eukaryotic and prokaryotic. Moving the function f x 2 units to the left will be the new function g x with the equation.

W x x53 4 x5 graph. Then graph the function. Shifting a graph horizontally A number adding or subtracting inside the parentheses or other grouping device.

Adding to the output of a function moves the graph up. Then graph the function. A column vector breaks down the translation into.

Converting genetic information into proteins has kept life in existence for billions of years. W x 2 x3 4x 2x3 8x. F x was translated to the left by 5 units to form g x Given the function f x x to g xx-5 if you look at the g x function you will see that 5 was subtracted from the abscissa x that is.

Subtracting from the output of a function moves the graph down. Transcription and translation are the two processes that convert a sequence of nucleotides from DNA into a sequence of amino acids to build the desired protein. Every point of the shape is moved in the same direction by the same distance.

Write gx and hx as transformations of fx. 2 The graph is reflected over the x-axis. Tx x 5 5 units down because k was subtracted Examine the equation for each parent graph table or equation labeled and its corresponding transformed graph table or equation labeled.

The original period divided by the constant b. Y axhk y a x - h k. So for example the function y sin2x will have period 2ˇ 2 ˇbtells you the number of the cycles of the sine function on an interval of length 2ˇThus the graph of y sin2xconsists.

у 3D x 51 3. Shift -- A rigid translation the shift does not change the size or shape of the graph of the function. A translation is a change in position resulting from addition or subtraction one that does not rotate or change the size or shape in any way.

W x x43 4 x4 Move 5 spaces left. Move 3 spaces down. Sketch a graph of each transformation of fx.

Qx 2x 3. Fx x Translated function. G xf x22 x22 -1.

Each of the seven graphed functions can be translated by shifting scaling or reflecting. The function v x x 3 4x.

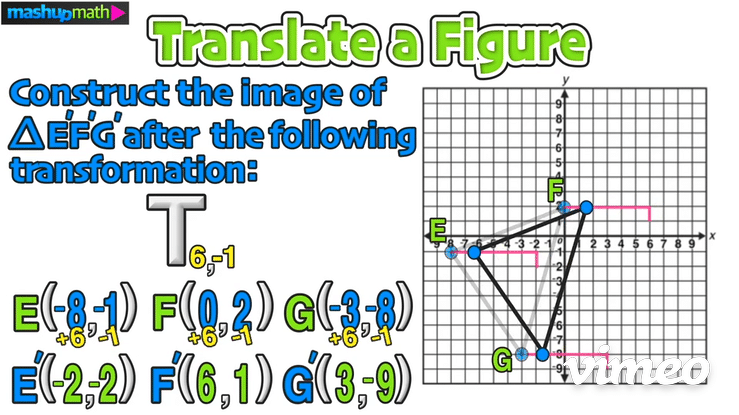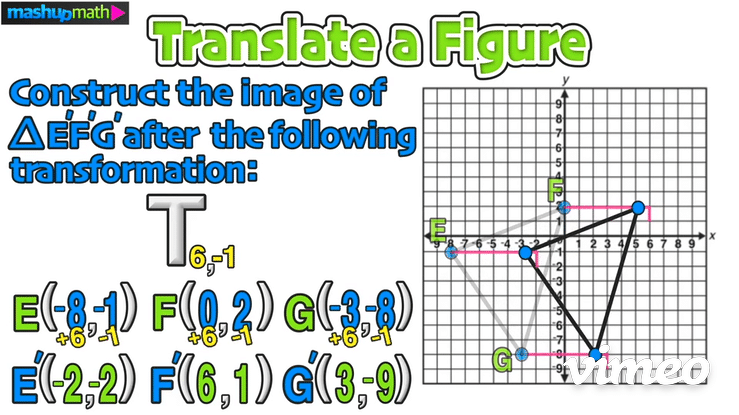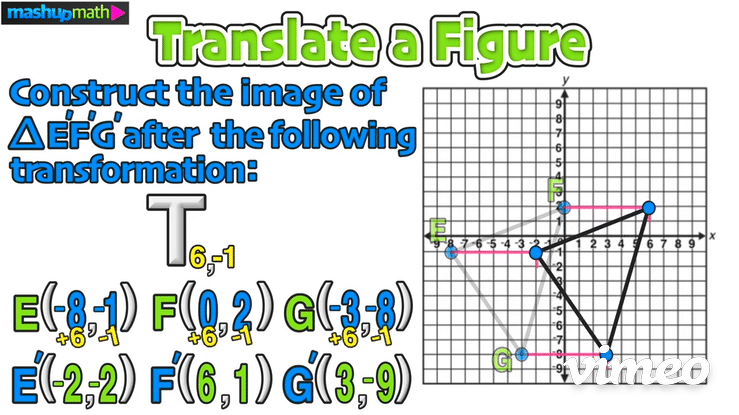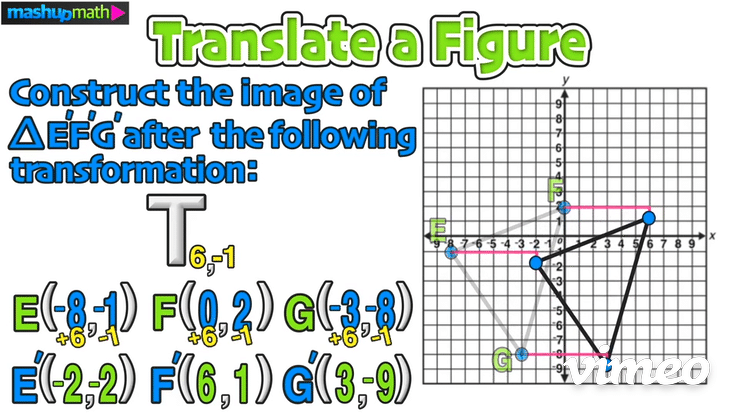
Geometry Translations Explained Examples And Extra Practice Mashup Math